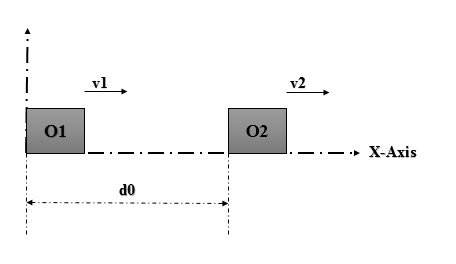
Consider two moving objectobjects along X-axis direction with different speeds and different initial starting points. Let the initial distance of the objects be d0 and v1 is greater than v2 i.e v1>v2.
physicPhysics says:
x1x1 = v1v1 * t + x01x01 = v1v1 * t
x2x2 = v2v2 * t + x02x02 = v2v2 * t + d0
ifIf we put x1x1 = x2x2 we obtain :
t = d0 / (v1v1 - v2v2)
and O1O1 because of its greater speed will eventually reach to O2O2.
butBut philosophy says othersomething else:
O1O1 in t_1=t1=(somthingsomething calculable) will reach to O2'sO2's current position but in this time interval O2O2 will move x_1=x1=(somthingsomething calculable) toward.
againAgain in the new situation O1O1 in t_2=t2=(somthingsomething calculable) will reach to O2'sO2's current position b but in this time interval O2O2 will x_2=be x2=(somthingsomething calculable) toward.
byeBy repeating this procedure t_ntn and x_nxn approaches to zeroszero but does not become zero, therefore O1O1 will never reaches O2reach O2.
How do you explain this?
thankThank you so much
best regards.
