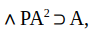Why does this proof hold?

Question

I'm currently reading Mathematics Without Numbers: Towards A Modal-Structural Interpretation by Geoffrey Hellman, and I'm on pages 26-27. It seems like Hellman is discussing opposition to viewing mathematical proofs solely through the interpretative structure of if-then. To avoid misunderstanding, I'll show you the passage first:

As already suggested, a categorical assumption to the effect that “ω-sequences are possible” is indispensable and of fundamental importance. Without it, we would have a species of “if-thenism”, i.e. a modal if-thenism, and this would be open to quite decisive objections, analogous to those which can be brought against a naïve, non-modal if-then interpretation. Consider the latter. Suppose it represents sentences A of arithmetic by means of a material conditional, say, of the form,

or some refinement thereof. Suppose also that, in fact, there happen to be no actual ω-sequences, i.e. that the antecedent of these conditionals is false. (This could be “by accident” as it were. For the sake of argument, do not insist upon Cantor's universe of sets as “necessary existents” (please!). Consider, instead, the stance of the “if-thenist” who seeks to avoid platonism.) Then, automatically, the translate of every sentence A of the original language is counted as true, and the scheme must be rejected as wildly inaccurate.

However, isn't it true that in a material conditional, even if the antecedent is false and the entire conditional is true, the truth value of the consequent isn't necessarily true? Why does negating the antecedent and affirming the conditional automatically argue that the consequent, an arithmetic statement A in this case, is true? I'm having trouble understanding what I'm missing here. The only unused clue seems to be that ω-sequences are not possible, which Hellman refers to in terms of numbers here, but this argument doesn't seem to involve that concept directly. What am I missing?

Answer 1

I don't have a copy of the book you reference, so I'm going by what you have quoted. Unlike some of the comments here and on MathSE, I think the author is doing more than just making a confusing reuse of the symbol A.

The author is criticising if-thenism in the context of the philosophy of mathematics. It might help to review what that means. Some mathematicians, particularly Russell, hold that mathematical theorems are necessarily true, but that no existential statement is necessarily true, i.e. nothing exists of necessity. However, some mathematical theorems take the form, "There exists an x such that...", so there is an apparent conflict.

The mathematical platonist is willing to bite the bullet and say that mathematical objects do exist of necessity and that their properties are necessary. But Russell is not sympathetic to platonism. He defended a version of logicism and claimed that mathematics is concerned only with implication relations. Russell's logicism has few defenders these days, but if-thenism persists as a variety of structuralism in mathematics. It is also consistent with some versions of formalism.

So, consider a sentence such as 2+2=4. A mathematical platonist would say simply that this is true, because numbers are real but immaterial things that float around in Plato's heaven, trying their best not to bump into the Form of the Good, and 2+2=4 is a fact about those things. An if-thenist prefers instead to say that when mathematicians assert 2+2=4 this is nothing more nor less than a claim that if the axioms of Peano arithmetic hold, then 2+2=4 follows. For the if-thenist, mathematics is all about saying: "if those axioms, then these theorems", but without commitment to whether the axioms are true.

Now for Hellman's criticism. He seems to be saying something like this: the if-thenist is mentally translating the sentence 2+2=4 into ∧PA2 ⊃ 2+2=4. And more generally, translating any arithmetical sentence A into a sentence of the form ∧PA2 ⊃ A. Hellman objects that this leads to A being trivially true in the event that the antecedent is false, because the implication just is the if-thenist translation of A itself.

This appears to be confusing two different approaches. The if-thenist doesn't care about whether the theorems of PA are 'true' or not. The if-thenist is committed only to the implication being correct, or that in any model of the theory, A will hold in that model. If there are no models of the antecedent, the implication is trivially true for any A, but that doesn't matter to the if-thenist.

At this point, it would be handy if I did have the book. It is possible that Hellman's argument rests on some more subtle consideration such that PA does not include the ω-rule and hence there might be implications within the language of PA that are rendered trivially true in the event of there being no actual ω-sequences. In which case, I'm not sure exactly what is the significance of his emphasised, "Suppose also, in fact, there happens to be no ω-sequences".