3

Dialetheism asserts that there are sentences that are both true and false, e.g. the Liar. This seems to, quite obviously, go against the law of non-contradiction (LNC), and indeed Priest seems to agree.

Dialetheism is the view that there are dialetheias. If we define a contradiction as a couple of sentences, one of which is the negation of the other, or as a conjunction of such sentences, then dialetheism amounts to the claim that there are true contradictions. As such, dialetheism opposes—contradicts—the Law of Non-Contradiction (LNC), sometimes also called the Law of Contradiction. - SEP entry on dialetheism as written by Priest

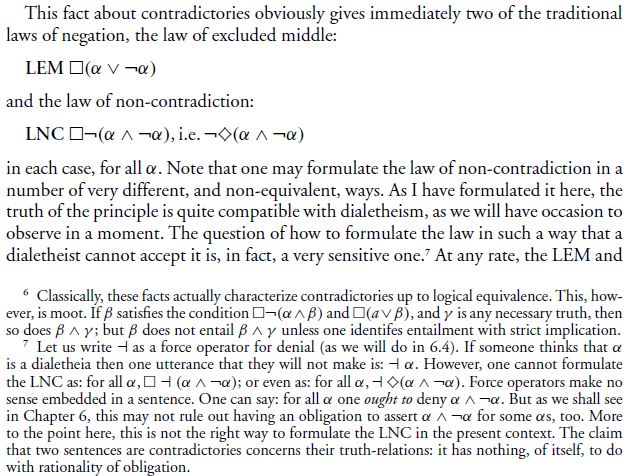
However, the LNC is actually valid in Priest's logic of paradox (LP):

...the law of noncontradiction. One must be careful here, however, about what exactly this means. What it does not mean is that the formula ¬(P∧ ¬P) is not logically valid (i.e., true in all interpretations). Indeed this formula is valid in the semantics of LP. What it does mean is that we must come to accept some formulas of the form P∧ ¬P, since some of these are indeed true. - Logic of paradox revisited

I am confused. How can the LNC be valid in LP if dialetheism is supposed to reject it? I take it from above that by being valid, Priest meant that the LNC is provable from no premise, and thus is a logical truth as far as LP is concerned? (If the LNC is provable only from a set of premise, that doesn't seem very useful as a law, so I doubt that's the case)

I can find no such proof in Priest's Logic of Paradox paper; I tried looking for a more detailed explanation in In Contradiction and Doubt Truth To Be a Liar, but I can't find anything except a small section (attached at the bottom).

Could anyone clarify what form of the LNC exactly does Priest oppose, and point me to the relevant literature please?

Excerpt from Doubt Truth To Be a Liar: enter image description here enter image description here

Improve this question
5
  • See LP (Priest's Logic of Paradox): One way of presenting the semantics for LP is to replace the usual functional valuation with a relational one. The binary relation V, relates a formula to a truth value: V(A,1), means that A is true, and V(A,0), means that A is false. A formula must be assigned at least one truth value, but there is no requirement that it be assigned at most one truth value." Negation swaps truth values (as usual) and thus A or not-A is always true, because in every case one of the two alternatives is true.
    – Mauro ALLEGRANZA
    Commented Jan 22, 2021 at 18:13
  • @MauroALLEGRANZA Isn't A or not A the law of excluded middle instead? Do you mean when DeMorgan is applied, from A or not A we can get ¬(A∧ ¬A)?
    – Constantly confused
    Commented Jan 23, 2021 at 13:28
  • 1
    Note that in inconsistent classical systems ¬(P∧ ¬P) is still derivable, so they too "accept" non-contradiction in this sense. What dialetheists reject is not the first order law ¬(P∧ ¬P), but rather its meta-version: either at most one of P and ¬P is derivable or any formula is derivable (by explosion). Hence some P∧¬P are derivable, but not for all P. Their logical system is neither consistent nor inconsistent, it is paraconsistent, which is not an option for classical logical systems.
    – Conifold
    Commented Jan 24, 2021 at 1:42
  • @Conifold Thank you, so for the Liar sentence L, (L∧ ¬L) would be true in LP but so will ¬(L∧ ¬L); but for an ordinary sentence P (e.g. 'Socrates is a man') either P or ¬P is true in LP, and thus ¬(P∧ ¬P) is true in LP? So (L∧ ¬L)∧¬(L∧ ¬L) is itself a dialetheia? But ¬(P∧ ¬P) is not dialetheia as long as P is an ordinary, non-Liar sentence?
    – Constantly confused
    Commented Jan 24, 2021 at 15:54
  • 1
    Basically, yes, but it is not restricted to the Liar sentence. There are true contradictions, a.k.a dialetheias, that are true along with their negations (like the Liar), and ordinary sentences that are only true or only false. And a change in derivation rules to block explosion, so P∧ ¬P -> Q is the first order law that they do reject, see SEP, Dialetheism.
    – Conifold
    Commented Jan 25, 2021 at 2:00

1 Answer 1

Reset to default
2

I believe that it is not so much a case of dialetheism rejecting a form of LNC, but rather a case of where LNC is applicable.

For the dialetheist, the Liar sentence has a literal meaning, but it has no content. It does not express a proposition. They say, don’t be fooled by the phrase “This statement”. For example, “The number that is one less than itself” does not describe a number.

As a sentence that is not a propositional statement, they argue that LNC does not apply to the Liar sentence. It is then a straight-forward argument to derive a vacuous biconditional from the Liar sentence - Liar if and only not(Liar).

Improve this answer
3
  • Would you mind pointing me to the relevant literature please? I don't think this is the position Priest takes because he explicitly said in 'What's so bad about contradictions?' that contradictions do have content (p.30 in 'The Law of non-contradiction', edited by Priest et al)
    – Constantly confused
    Commented Jan 24, 2021 at 15:12
  • @DanielMak My "lazy" (unreferenced) answer is a paraphrase of what I recall from reading the text which you cite (The Law of Noncontradiction edited by Priest, et al.). I read it while I was still at uni, and this was the explanation of the Liar sentence that made the most sense to me at the time - namely that LNC applies only to propositional statements. I cannot recall whose paper provides this explanation, but I suspect that it was not Priest's introductory paper "What's so bad about contradictions?". Perhaps the content that Priest identifies is outside of a propositional calculus.
    – nwr
    Commented Jan 24, 2021 at 20:04
  • ... further to my comment above, is it possible that the content which Priest identifies is in the paradox derived from the Liar sentence ( Liar and not(Liar) ), rather than in the Liar sentence itself.
    – nwr
    Commented Jan 24, 2021 at 20:50

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .