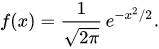The physical universe does not adhere to Mathematical principles, but instead, Math was inspired by the physical universe.
If I have an apple and you give me an apple, I can think how they both look the same and I can say, 1+1=2. Math evolved like this and mathematicians tried extending it beyond what we could test in real life, and then later tests in real life confirmed what they had already theorized.
For example, the imaginary number i was made up to solve the square root of negative one problem. And many years later, in Quantum Mechanics, I'm pretty sure that the number i was confirmed to exist in nature as a "real" number, despite the number being called imaginary, because mathematicians at the time thought they were making it up.
Now consider Physics, which began as philosophy of nature, people asking questions about nature.
When a large object is moving really fast, it appears to have more power to cause damage. If a small object is moving fast, it still has power, but less power. And if a big object is moving slow, it has power but less, and if a small object is moving slow, it has very little power or strength. This is where f = ma comes from. The mass TIMES the acceleration of the object directly relate to its force. Our initial observations appear to be backed by evidence, that there is a very specific relationship between mass and acceleration and force that can be expressed in math for clearness.
We don't have to use math to explain the universe, I can explain any physical phenomenon in English, but the good thing about math is that it is clear and objective. Which makes math a better tool for explaining things.
Math is a process of gathering facts and identities and giving them names so you can reference them later when it's appropriate. It is a model that was created to be clear, explicit, and objective, and this makes it very useful for describing relationships in the universe.
E = mc^2, this is very clear and easy to understand, there is no room for vagueness. It is describing a relationship regarding the proportion of mass and speed of light and how they equate to energy. An equation like this is more precise than using words.
The fact that the universe appears to be consistent, and that it appears to be exact, is what makes Math so useful in modeling the universe physically. Because many things in the universe exist as relationships to one another, direct relationships that are consistent and precise, this kind of thing is what math is good at modeling and describing.
In summary I think math applies to nature because Math is a useful framework for explicitly defining logical relationships between different things, and creating a consistent dogma that can be extended or used when applicable. If there are no contradictions in physics, then establishing mathematical rules should apply and therefore be able to be extended to discover new answers.
Math is useful for defining objective facts which always hold true, and therefore you can consider previous facts when defining new facts. And you can consistently rely upon those relationships when performing precise calculations, because the universe naturally appears to also exist in this way. It is consistent and objective and does not contradict itself.