Is there such a thing as absolute proof?

Question

Can you prove, absolutely and 100%, that something is true, in the field of philosophy? It always seems you can go a layer down, and find another question, almost endlessly.

Reference

Development of Proof Theory

Answer 1

There are two categories of things that can be proved in philosophy:

• That a thinking thing exists;
• The trivial truths of logic.

I'll cover these in order. In fact, there are philosophical arguments you'll find against them both. The basic idea that 'a thinking thing exists' comes to us via the Ancient Greeks but became widely known and was made popular by Descartes in his Meditations.

In this work he doubted everything he possibly could until he reached a base, the truth of which he could be absolutely certain. He thought it important to have a solid foundation to build his philosophical system on.

Archimedes used to demand just one firm and immovable point in order to shift the entire earth; so I too can hope for great things if I manage to find just one thing, however slight, that is certain and unshakable.

(Unfortunately he very quickly lost his way and went from this solid foundation to a very questionable argument for the existence of God.)

Cogito ergo sum ("I think therefore I am") is the famous phrase from Descartes' Meditations. "I think therefore I am" is a stronger statement than "A thinking thing exists" so I have put the second forward for this answer.

It is in the class of truths that are self-evident. Thinking about it proves its truth. In philosophy we can't do physical experiments to disprove our theories so we need to rely on thought experiments instead. This is an example of a thought experiment. I can't conceive of any logically possible way of this being self-contradictory, i.e. false. By simply doing that thinking I have proved the proposition's truth.

---

The second class of provable things are the trivial truths of deductive logic. I'll divide this into two parts:

• The Laws of Thought - axiomatic laws that we should agree on before we can start discussing philosophy.
• The truths of propositional logic - these follow after we set up an axiomatic system.

I'll cover these in turn, very briefly. I'll leave others to tear them down.

The Laws of Thought as a collection are attributed, like so much in philosophy, to Aristotle. They are:

• The Law of Identity - an object is the same as itself - (A ≡ A).
• The Law of (Non)-Contradiction - "the same attribute cannot at the same time belong and not belong to the same subject and in the same respect"¹ - ¬(P ∧ ¬P).
• The Law of the Excluded Middle - "it will not be possible to be and not to be the same thing... there cannot be an intermediate between contradictories, but of one subject we must either affirm or deny any one predicate."² - (P ∨ ¬P).

There are arguments against each of these.

Propositional logic is a simple formal system. We define what is and isn't true via truth tables before we enter into discourse about it.

A simple truth, by definition, in propositional logic is found in logical conjunction. Here's the truth table (from wikipedia):

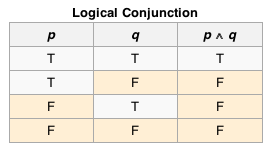
If both of its operands (p, q) are true the conjunction of them (p ∧ q) is also true.

---

¹Aristotle, Metaphysics. Aristotle claimed this as the most secure and unshakable of all principles.

²Ibid.

Answer 2

Philosophy is generally predicated on, and perhaps more about, asking questions rather than finding answers. It's a search for wisdom, not truth. The only thing that all philosophers would all agree exists, besides themselves perhaps, would be questions. And sometimes, philosophers will pretend that even those don't exist. So, philosophy doesn't, as a whole, assume that any fundamental rules exist upon which to build "proven" answers.

Proof is a concept in mathematics, and mathematics is in some ways a formalized version of philosophy that HAS acknowledged the existence of fundamental rules (axioms). It is also a concept in legal systems, where again, you have formal systems that have fundamental rules (laws).

For fun, read about Gödel's incompleteness theorems. Even formal systems with fundamental rules have problems.

Note: This answer assumes you're asking whether it is possible to prove any SINGLE thing in philosophy. If you are instead asking if it's possible to prove ANYTHING, well, that's a different question. I'm sure SOME people would say you can.

Answer 3

It depends.

Consider the philosophers of science, their struggle is to define the nature of a fact or the "proven true" statement.

As there has been no final conclusion in the generational debate between Carnap, Popper, Kuhn, Lakatos, Feyerabend, and others, it would be difficult to apply their understandings to the domain of philosophy as a whole.

However, We can state that Carnap's refutation of validation:

The first thesis of physicalism may then be regarded as a new formulation of the principles of empiricism: (1) Statements are to be regarded as scientifically meaningful only if they are in principle intersubjectively confirmable or disconfirmable. If a statement, by the very interpretation imposed upon it, is in principle incapable even of the most indirect sort of intersubjective test, then though it may have meaning of a purely logical sort, or may be significant in that it carries pictorial, emotional or motivative appeals, or may even be testable in an exclusively subjective manner, it cannot be accepted as an answer to a scientific question. The phrase "in principle intersubjectively confirmable or disconfirmable" should be understood in the most liberal manner. The sort of indirect testing of assertions here allowed for includes of course the testing of only partially interpreted postulate systems. It countenances as scientifically meaningful, statements about the most remote, the most intricately concealed or difficult to disentangle states of affairs. It includes statements about unique and unrepeatable occurrences, if only they are of a type that places them within the spatio-temporal-nomological net which itself has an intersubjective confirmation base. (2) Statements are to be accepted as scientifically valid only if they are sufficiently highly confirmed by in principle intersubjectively available evidence. The precise meaning of "sufficiently highly confirmed," as well as the exact explication of "degree of confirmation," "inductive probability," or "evidential support" need not be discussed in the present context.

His use of "spatio-temporal-nomological net" restricts our knowing to confirmation as our perceptions-of-world are anchored temporally to the here-and-now. As we cannot perceive or predict the entire totality of the universe, we cannot declare that any statement is absolutely true or false, even in science, much less in the far harder to test reaches of philosophy.

Answer 4

Short answer: it all depends on your meta-theory

Long answer (from the Preface of D. Hofstadter's I am a strange loop):

It seems to me that many philosophers believe that, like mathematicians, they can actually prove the points they believe in, and to that end, they often try to use highly rigorous and technical language, and sometimes they attempt to anticipate and to counter all possible counter-arguments. I admire such self-confidence, but I am a bit less optimistic and a bit more fatalistic. I don’t think one can truly prove anything in philosophy; I think one can merely try to convince, and probably one will wind up convincing only those people who started out fairly close to the position one is advocating.

Answer 5

Formal logic is a branch of philosophy, and yes, you can certainly prove that a given argument is valid. Other branches of philosophy, of course, have bigger issues with provability. Aesthetics, for example, doesn't lend itself to objective proof very well. Ontology and epistemology can at times shade into science, although of course many views of the philosophy of science reject that anything can be proven absolutely.

That said, your question itself is open to philosophical inquiry; certainly many philosophers have believed that they were proving things conclusively; you'd never convince Descartes that he did not in fact prove to himself that he existed, and many of Aristotle's ideas were held to be truths for many centuries.

Answer 6

We would do well to keep in mind Dummett's notion of verification-transcendent truth, or what realists might commit themselves to with respect to either metaphysics/epistemology or logic (that some true things can't be known, or that some logical truths can't be proven).

Answer 7

Well, depends on what one means by an absolute proof. Agripa's trillemma states that every attempt to completely justify a proposition terminates into one of three possibilities:

1- arbitrariety/indemonstrables. (Basically, we have a proposition A, which is justified by B, and B by C, and so on until we finally reach a proposition which requires no further justification, such that is true by self-evidence and by assumption, being an indemonstrable, serving as a foundation for all other propositions. This is the most plausible hypothesis, with the only problem that it is coined as being arbitrary because there is no way to properly select whether it is true or not because it is self-evident and pressupositional, so there can be no higher principle to judge whether it functions or not. This option comes from a finite chain of justifications, which can also be called as foundationalism.

2- infinite regress. (All propositions require another proposition to be justified. So we do the same process as in 1, except we do not end at any proposition, there is no indemomstrable. This is what happens when we have a non-finite chain of justifications. There is no satisfactory notion of a complete justification here, for no proposition suffices for this role, given the very definition of this chain of justifications.)

3- circular reasoning (in the other two cases, the chain is such that it is non-recursive. That is, once a proposition is justified, it will never appear back again in the chain of justifications, such that it will never justify any proposition as we go down the chain. So, for example, if we have a proposition A that is justified by B, it cannot be the case that B is justified by A, or if B is justified by C, then it cannot be the case that C is justified by A or by B, and so on. We never return to any previous member of the chain. But circular reasoning is a recursive chain, so it might be the case that A is justified by B, but then we see that B is justified by A. This is the worst end to an attempt to completely justify a proposition.)

The "absolutely proven" propositions would be more possible in the first option. So we can have a proposition that follows necessarily of an indemonstrable, such as the statement "p → ¬¬p", which follows necessarily from the law of non-contradiction, as p ∧ ¬p is prohibited given this laws. However, there are people who might reject the law of non-contradiction, such as dialetheists, who defend the existence of dialetheias, which is a term used to refer to true contradictions. In classical logic, all contradictions are false, so if we to put a truth table of p and p ∧ ¬p, it would go like this:

As you can see, the truth value of the contradiction is always false. There are other indemonstrables aside from the LNC, such as the law of excluded-middle, which can also be called as the principle of bivalence. Basically, either a proposition is true or it is false (p ∨ ¬p), so there are only two truth values available: T and F. This is also quite intuitive. The indemonstrables tend to be extremely intuitive (unsurprisingly), as they basically flourish from the very meaning of the propositions involved, which is why any proposition that violates them is rendered to be...well, meaningless. So, as a reply to the question, i would say that yes, absolute proofs do exist. But even if we are not talking about rigorous proofs based on first principles/indemonstrables, there's a truth which is just as evident, which is the notion expressed by the frase "cogito ergo sum". The very fact that i doubt undoubtably leads to the conclusion that i think, and this fact undoubtably leads to the conclusion that i exist, such that there can be no way for me to deny the validity of this inference. Even the most extreme position would be solipsism, which still embraces the truth of this notion above ALL else.

Answer 8

Can you name a field where anything is proved i.e finalised in every possible aspect? By their nature people seek simple things, like religion to promise them if they satisfy a criteria then they are gonna be ok. In philosophy an end result for anything is just an starting point for something else, it appeals to those who don't want just a yes or no answer but a 'why' as answer.

PS: as Joseph Spiros pointed out by refrencing Godel, the very nature of proofs are questioned in philosophy.

Answer 9

Proving any statement is true or probably true is impossible, unnecessary and undesirable. This is true whether the statement is deemed to be philosophical or not. If you assess ideas using argument then the arguments have premises and rules of inference and the result of the argument may not be true (or probably true) if the premises and rules of inference are false. You might try to solve this by coming up with a new argument that proves the premises and rules of inference but then you have the same problem with those premises and rules of inference. You might say that some stuff is indubitably true (or probably true), and you can use that as a foundation. But that just means you have cut off a possible avenue of intellectual progress since the foundation can't be explained in terms of anything deeper. And in any case there is nothing that can fill that role. Sense experience won't work since you can misinterpret information from your sense organs, e.g. - optical illusions. Sense organs also fail to record lots of stuff that does exist, e.g. - neutrinos. Scientific instruments aren't infallible either since you can make mistakes in setting them up, in interpreting information from them and so on.

We don't create knowledge (useful or explanatory information) by showing stuff is true or probably true for reasons so how do we create knowledge? We can only create knowledge by finding mistakes in our current ideas and correcting them piecemeal. You notice a problem with your current ideas, propose solutions, criticise the solutions until only one is left and then find a new problem. We shouldn't say that a theory is false because it hasn't been proven because this applies to all theories. Rather, we should look at what problems it aims to solve and ask whether it solves them. We should look at whether it is compatible with other current knowledge and if not try to figure out the best solution. Should the new idea be discarded or the old idea or can some variant of both solve the problem?

See See "Realism and the Aim of Science" by Karl Popper, especially chapter I and "The Retreat to Commitment" by W. W. Bartley III.

Answer 10

To prove something true, you need to 1) know truth, 2) to agree about it and 3) to verify it on a system.

1. The only essential truth we know is causality.

Why 1+1 => 2? Because if you put an apple in an empty bag, then add another, and you count the apples, you will find two apples. The same happens if you-hit-your-head-against-the-wall => you-feel-pain. This mechanism of action and reaction is called causality. We learn the action-reaction mechanism before being born and along all our lives. When something breaks causality, we feel pain, anguish, fear. Imagine you bash your head against the wall, but you don't feel nothing. Imagine you lose a loving one. Imagine you see an UFO or a miracle. All this events break the causality rules in our head and we lose the sense of reality. The only reality we accept is the one we understand, and that is the one which follows the causality mechanism.

A proof is the application of the causal rule over a system.

2. Causality is an objective knowledge.

Therefore, we all know the mechanism, and we can share thoughts. You will probably agree that if you speak to a rock, it will not answer as a person would do. Therefore you and me can find the same proof. If we don't agree with causality, proof is impossible. Objectivity about causality is probably the base of all science. There is no deeper truth (for now).

3. Proof is finding that a consequence is related to a cause by a system.

As you and me 1) know the causality mechanism, and 2) agree about it, finding a proof is making a causal relationship between a cause and a consequence. Then, we can agree that sunlight makes plants grow (causal rule: sunlight-over-a-plant => plant-grows). Then, we can set a system plant, send the sunlight input (cause or action) and verify growth (reaction). Finding the system that relates action to reaction, or finding specific exceptional reactions can be difficult. But sometimes we find them and agree and reach a proof.

Answer 11

If you include the logical sciences as part of philosophy, then yes you can, but only with deductive logic. But like mathematics, for any proof you must begin with assumed truths (premises), and build from them.

If you mean whether can you prove something from nothing? Well I have no idea.

Answer 12

Believe in nothing, consider everything. I think philosophy's most valuable insight is the realization that nothing can be known with absolute certainty. Obviously we want to base our decisions on what seems most logical and practical but i think it's very important to always approach things with some level of caution and uncertainty in the back of your mind. You have to accept the fact that human beings are not capable of complete understanding. It's not always about what you see, but what you don't see. The only truth in our world is that those who claim to have found an infallible truth are not to be taken seriously.

Answer 13

Ultimately, nobody can prove anything with complete confidence, no matter the method used. See Underdetermination.

Answer 14

I think this question is a good opportunity to consider some anti-skeptical arguments, that have interested me for a long time. A few different people have made interesting claims that radical uncertainty may be self-defeating. Also, on a practical level, it does seem that there are some forms of knowledge that are consistently reliable. From an empirical perspective, it can seem like the task at hand is to explain why the world is not beyond comprehension, even though a priori we often feel inclined to conjecture that it is: the reliability of some forms of knowledge is a data point in conflict with skeptical aspersions towards certainty, in other words. Thus, the easy claim is that “we can’t truly know anything”, without any justification for this claim: taking it as a default simply because the person in that moment can’t think of any way there could be (an availability bias or the “philosopher’s fallacy”). The hard claim is that we are wrong, and there is actually a way to know something with a high degree of confidence or certainty; and we must do difficult theorization, as in all other scientific fields, to determine precisely what it is or may be.

(To be updated and continued in the course of time.)