Mauro's answer is complete and authoritative, and Wikipedia's article on subalternation is technical, but illuminating. Let's start with an example in natural language:
An example of a subalternation is "If all squares are quadrilaterals, then some quadrilaterals are squares."
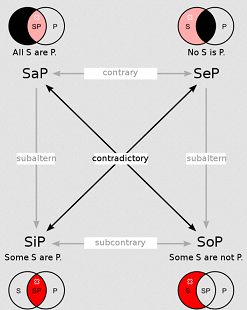
All we've done is drawn a more particular conclusion from the original proposition. What is important is that the truth follows from form or syntax, not content or semantics. Thus, it is of the form:
∀s Qs ⊨ ∃s Qs
Where "∀s" means "all squares", "∃s" means "there exists some squares", and "Qs" means "squares are quadrilaterals". The symbol "⊨" can be read as "always entails" and means that there are no exceptions to the rule. Put together: All squares are quadrilaterals always entails some squares are quadrilaterals. In terms of the Venn diagram, this means that if all members of one circle are in another circle, some portion of those members must be in the circle.
Ultimately, it's one type of relationship that can be found to inhere to the square of opposition. You ask:
Is the subalternation relationship between superaltern and subaltern a derivational one between two propositions, or implicational one between component propositions in a compound proposition?
Thus, the superaltern always implies the subaltern, but not vice versa. If a conclusion and antecedent imply each other, then the implication is a contraposition and is qualified as biconditional. For instance:
"Some quadrilaterals are squares does not imply all quadrilaterals are squares" is true.
Indeed, kites are not. The biconditional is thus false.
"Some quadrilaterals are squares implies all quadrilaterals are squares" is false.
Material implication is a type of logical consequence, and a derivation in the language of logic is a proof. From the article on formal proof:
In logic and mathematics, a formal proof or derivation is a finite sequence of sentences (called well-formed formulas in the case of a formal language), each of which is an axiom, an assumption, or follows from the preceding sentences in the sequence by a rule of inference. It differs from a natural language argument in that it is rigorous, unambiguous and mechanically verifiable.

