In 2001: A Space Odyssey, David Bowman approaches the monolith orbiting Jupiter and transmits a series of radio pulse to indicate that he's intelligent: 1 pulse, then 2, 3, 5, 7, 11, 13, 17; and then repeated.
He did that because supposedly, repeating prime number sequences do not occur in nature.
William Dembski also asserts that receiving a sequence of prime numbers of clicks by radio would be de facto evidence of life.
Is it?
You still need null hypothesis testing. (And systemic discouragement of P-hacking.) Any finite list of primes can be generated by any random system that generates number sequences: if you sample enough radio static and encode it into strings of 800 whole numbers in base 18 for some reason, roughly one out of every 18^800 of those 800-digit numbers will be 12357BDH repeated 100 times in a row.
If you take a few thousand samples and you get one signal that's 12357BDH repeated 100 times in a row, that's pretty close to proof positive of intelligent design. (Probably the unregistered human ET enthusiasts on the hillside a few miles away from your radio telescope, not aliens, but intelligently designed nonetheless.) If you took 18^800 samples and you got one signal of 12357BDH repeated 100 times in a row, you haven't actually found anything interesting.
Edit: The probability is one in 18^800 (or on that order of magnitude, if the static is not a perfect random number generator) and not 800^18. That changes the answer's conclusion, since it is such extremely small probability.
There is reportedly something about cicada cycles being prime-numbered that is important/relevant (see in the SEP here), but if someone thinks a priori that such numbering is evidence of intent, then one would have a motive to think that cicada cycles were intentionally set up that way, etc.
Another thing to consider is that the sequence of prime numbers is but one of arbitrarily many "interesting" sequences of numbers (see Wikipedia here; the source for that is a text with hundreds of thousands of sequences listed). Sometimes, physical manifestations of e.g. the Fibonacci sequence just fall out of the pertinent background mathematics. So there are some uncertain odds of various processes "following" this or that sequence, effectively by happenstance. There are, that is, 2ℵ0 n-sequences with countably many stages; which is uncountably many, in theory. So in theory, there are uncountably many n-sequences, and it is not rigorous to cite just intuitions about design-suggestive sequences, to make the pertinent case. (If any intelligible sequence could provide for evidence of conscious, volitional intelligence and not some automatic ordering principle of things, then we would trivialize our evidence base, here, it seems.)
Prime numbers can be "computed" by physical processes, when those physical processes logically resemble trial division, the sieve of Eratosthenes, or so on. For example, this paper on arXiv describes a system where interference of light passing through diffraction gratings is able to compute prime numbers; this one describes an optical effect which distinguishes primes from composite numbers.
Diffraction patterns can be produced by narrow holes in a static object, or by objects close together with narrow gaps between them. The more complex an arrangement of slits needed to produce a diffraction pattern, the less likely it is to occur in nature by chance; but to get the primes up to 23, you only need to sieve out the non-trivial multiples of 2 and 3. By my reading of the first paper linked above, that would take just two objects, with two and three slits respectively, in such an arrangement:
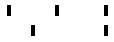
If I saw a pattern like this in nature, I wouldn't assume it must have been configured that way by a designer. But if you shine a strong light through the slits, then on the other side you would see bright spots spaced out like the sequence of primes in your question.
To draw a more general conclusion, it is not enough to calculate the probability that something we observe could happen "by chance" with the assumption of uniform randomness, because things in the universe obey physical laws which cause them not to be uniform. True, if you roll 18-sided dice then there is a 1 in 188 chance of seeing your particular sequence of 8 numbers, and that's not very likely. But the universe doesn't roll 18-sided dice, it obeys physical laws, and so we are heavily biased towards observing things which are possible according to those laws. And those possibilities don't form a uniform distribution. If we see something which seems to be cosmically unlikely according to the distribution we expect from the laws of physics, that doesn't tell us a supernatural designer did it, it tells us we are thinking according to the wrong probability distribution because we don't know all the laws of physics (or because we cannot compute all possible consequences of the laws we do know).
The universe may have been purely random at some initial point in time, but as time progresses, things get further apart due to the expansion of space, things fall together due to gravity, radioactive elements decay, and living things die. If you leave an instance of Conway's Game of Life running for trillions of iterations, there are certain patterns which are very likely to appear simply because those are the patterns which are able to still exist after such a long period of time. The same goes for real life.
Making an analogy to intelligent design, the probability of ~1028 atoms randomly being arranged to form a human body is impossibly small. But humans are not created by random arrangement of atoms, we are created by sexual reproduction: "descent with modification". Without any designer or intentionality, populations of anything (even data or algorithms) formed by descent with modification, subject to some selective pressure, will tend to either result in members which are adapted to those selective pressures, or otherwise die out completely. That is a mathematically inevitable consequence of survivorship bias plus time.
There is nothing stopping any natural process from producing something random that can be represented in numbers. And as such, any such process can coincidentally produce any finite sequence of prime numbers, with decreasing probability the longer the sequence ought to be.
Other than such coincidences, here is as example: https://www.princeton.edu/news/2018/09/05/surprising-hidden-order-unites-prime-numbers-and-crystal-materials
A new analysis by Princeton University researchers has uncovered patterns in primes that are similar to those found in the positions of atoms inside certain crystal-like materials. The study, “Uncovering multiscale order in the prime numbers via scattering(Link is external),” by Salvatore Torquato, Ge Zhang and Matthew de Courcy-Ireland, was published in the Journal of Statistical Mechanics: Theory and Experiment on Sept. 5
However, observation of prime numbers or other patterns could still be a way to detect an attempt at communication by an intelligent agent if something is a likely communication channel. The prime numbers are not necessarily more suitable than other sequences or patterns. Also see the https://en.wikipedia.org/wiki/Pioneer_plaque
You seem to be mixing two different questions that have different answers.
I'm asking if it's possible for a sequence of prime numbers to be generated in physical reality.
But then you give an example:
David Bowman approaches the monolith orbiting Jupiter, and transmits a series of radio pulse to indicate that he's intelligent: 1 pulse, then 2, 3, 5, 7, 11, 13, 17; and then repeated
So yes, clearly it can physically happen. Even if the movie doesnt show those pulses, its trivial to physically create them yourself based on this description you've given. I just tapped that pattern on my desk, making it physically exist (feel free to do the same if you need to prove it to yourself).
Your title question asks
Do prime numbers ever occur in nature? That is, would their occurrence be de facto proof of intelligence?
By asking the question like this, it seems like you start from the conclusion that intelligence is not "natural", so this line of argument can't be used to prove design, when design is already assumed as a premise.
There's still the question of whether anything non-intelligent can produce prime sequences (which other answers address), but I think it's important to recognize how that's not the same as asking if anything physically creates those sequences (unless you make the strange assumption that anything that ever interacted with an intelligent being is now non-physical).
I think there is some validity in the claim that a sufficiently long sufficiently interesting string of data presents itself that this is "proof of intelligence." The devil is in the details of course. As the accepted answer points out, a uniform random sequence of values will eventually produce all "interesting strings of data." It points out that we can do null hypothesis testing.
However, the null hypothesis is more complex. We see really interesting patterns in nature, such as this picture of a cone snail camouflage which can be generated using a simple cellular automata called Rule 30. A nearby automata, Rule 110, is proven to be Turing complete!
So we have to assume there are more interesting sources of data out there besides unprocessed randomness.
And this finally leads to the crux of the argument I'd like to make. At some point, a sufficiently long sufficiently interesting string of data becomes highly unlikely to be created by known processes that we do not consider to be intelligent. The probability of it being intelligent goes up. At some point the probability becomes high enough that we have to admit that our definition of intelligent isn't very precise. We regularly have disagreements as to whether particular animals qualify as intelligent or not. So at some point, the uniqueness of the data we receive should become so compelling that we have to go back and admit that we don't really understand what it means to be intelligent, and that maybe this is a reasonable definition of intelligence.
This would be very akin to Alan Turing's argument in Computing Machinery and Intelligence. In this paper, he does not argue that generating a sufficiently spectacular set of inputs demonstrates intelligence, but rather argues that "is this intelligent?" is not the best question to be asking. There may be a better question which is more nuanced and subtle.
There is nothing impossible in the generation of prime numbers given that the attachment we have to it is purely personal. The probability of generating a prime sequence is the same as the sequence 1 2 3 4 5 6 7 and this breaks no laws whatsoever. It is merely very improbable.
Now, you cannot infer design until you can show that that very designer is more probable than even the most improbable of improbable things! It doesn’t matter how improbable the prime number sequence is. The designer’s very existence must be more probable for you to conclude that he exists. It doesn’t even serve as evidence, much less “proof”, until that is established.
And despite our wants, we still have no evidence that other intelligent, conscious entities exist except us. Pointing out the vast expanses of the universe isn’t evidence. And we still don’t know how probable it is for intelligent life forms to come about through evolution, nor do we still understand the probability of the origin of life.
