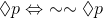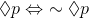Are statements in modal logic true or false?

Question

A bit new to modal logic.

I believe that it is the case if we say "It is possible that P is true in world w", then this statement is "true". This is not the same as saying P is true. Just that P is possibly true.

If we were to say "It is possible that P is true in world w and impossible that P is true in world w" this is a contradiction, so this statement is false.

Is that correct?

Wondering what the best way to formally state this would be. Can these types of statements be proven like in classical logic? I assume we can prove the truth of modal statements by constructing a proof and a theorem. However, in doing so, we don't actually prove whether P is true in a possible world and construct a truth table to show this is the case.

Edit:

More specifically, to state the above formally, what are the truth values of the following statements? True, false, or do they not have a truth value?:

The second statement looks like a contradiction, so it should be false.

Answer 1

Yes this is correct. A modal statement "it is possible that P" has a truth value that need not be that of P, because the latter depends on whether P obtains in the actual world, whereas the former depends on whether P obtains in at least one possible world.

Note though that possible world semantics is just a way to analyse modal statements. One must distinguish the semantics (a mathematical construction that represents what a statement is talking about) and the syntax (the form and construction rules of statements). Modal statements usually don't refer to specific worlds, they only contain "possible" and "necessary" operators, so I would have framed the question differently without mentioning specific worlds.

For example, your contradiction example could be "it is possible that P and it is necessary that not-p", which is contradictory.

There are indeed proofs in modal logic. You can either use deduction proofs using the axioms of modal logic (there are different axioms associated with different systems, but a common base) or natural deduction rules. Truth tables are the semantics of non-modal logic. Since the semantics of modal logic is different, you cannot use them: you need a possible world semantics.

Your two examples are as you say: the first is a logical truth and the second a logical contradiction. You don't even need to interpret the modal operator to know that, and you could have constructed contradictions that are specific to modal logic (such as <>p <=> []~p or p^[]~p)

Finally, modal statements have a truth value as a matter of logic, but it is more contentious whether or not they have a truth value as a matter of metaphysics (whether they correspond to something in the world). This depends on the kind of modality involved (nomological necessity, metaphysical necessity...), and some authors deny that they have truth values: they would be a mere way of talking.

Answer 2

The statements "Possibly P" and "P" are different statements. The rules of modal logic tell you how these are connected, but you should be careful not to let your intution about what these might mean interfere with following the rules.

To say "Possibly P and not possibly P" is just an instance of "Q and not Q" (just abbreviate "Possibly P" with "Q"), hence it is the same contradiction you are familiar with. Note that "Possible P and possibly not P" is consistent in many modal logics.

The problem with extending the truth table method to modal logic is that constructing the truth table for a propositional formula means that you check all possible models. For any propositional formula, there are only finitely many models that matter (the rows in the truth table). For modal logic, you need to concider how the worlds are connected (and how many there are). Thus, you find that a priori, you would need to check infinitely many models to ensure that a formula is always true.

You can still do proofs in a sequent calculus (or similar systems).